Finding the area of ​​a rhombus: formula and examples

Rhombus is a geometric figure; parallelogram with 4 equal sides.

Area formula

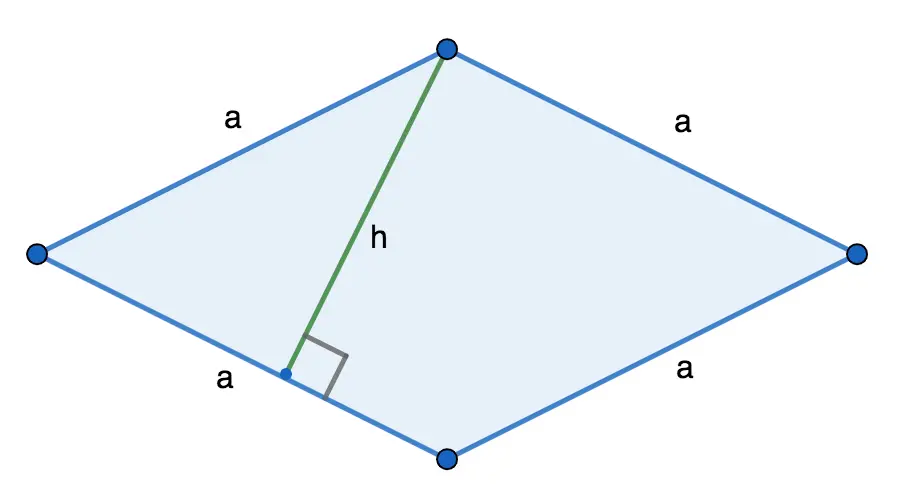
Side length and height

The area of ​​a rhombus (S) is equal to the product of the length of its side and the height drawn to it:

S = a ⋅ h

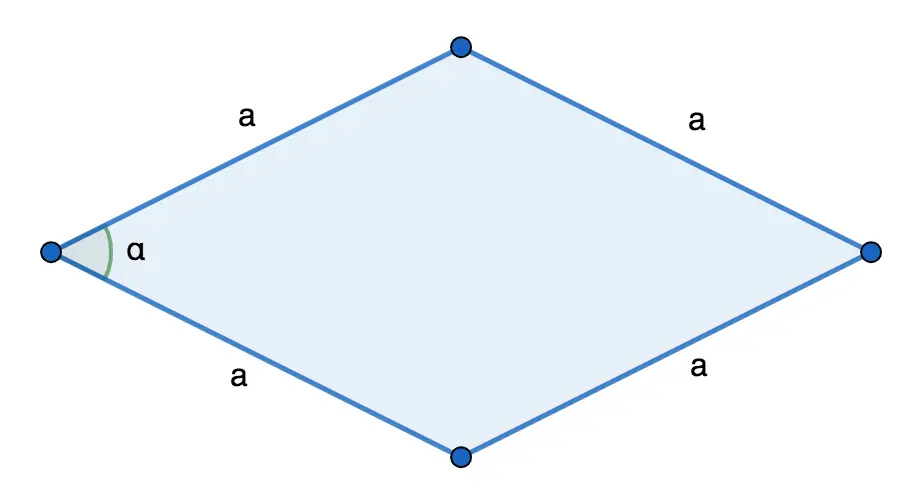
By side length and angle

The area of ​​a rhombus is equal to the product of the square of the length of its side and the sine of the angle between the sides:

S = a ² ⋅ without α

By the length of the diagonals

The area of ​​a rhombus is one-half the product of its diagonals.

S = ¹/₂ ⋅ d₁ ⋅ d₂

Examples of tasks

Task 1

Find the area of ​​a rhombus if the length of its side is 10 cm and the height drawn to it is 8 cm.

Decision:

We use the first formula discussed above: S u10d 8 cm ⋅ 80 cm uXNUMXd XNUMX cm².

Task 2

Find the area of ​​a rhombus whose side is 6 cm and whose acute angle is 30°.

Decision:

We apply the second formula, which uses the quantities known by the conditions of setting: S = (6 cm)² ⋅ sin 30° = 36 cm² ⋅ 1/2 = 18 cm².

Task 3

Find the area of ​​a rhombus if its diagonals are 4 and 8 cm, respectively.

Decision:

Let’s use the third formula, which uses the lengths of the diagonals: S = 1/2 ⋅ 4 cm ⋅ 8 cm = 16 cm².