What is a regular pyramid: definition, types, properties

In this publication, we will consider the definition, types (triangular, quadrangular, hexagonal) and the main properties of a regular pyramid. The presented information is accompanied by visual drawings for better perception.

Definition of a regular pyramid

Regular pyramid – this, the base of which is a regular polygon, and the top of the figure is projected into the center of its base.

The most common types of regular pyramids are triangular, quadrangular and hexagonal. Let’s consider them in more detail.

Types of regular pyramid

Regular triangular pyramid

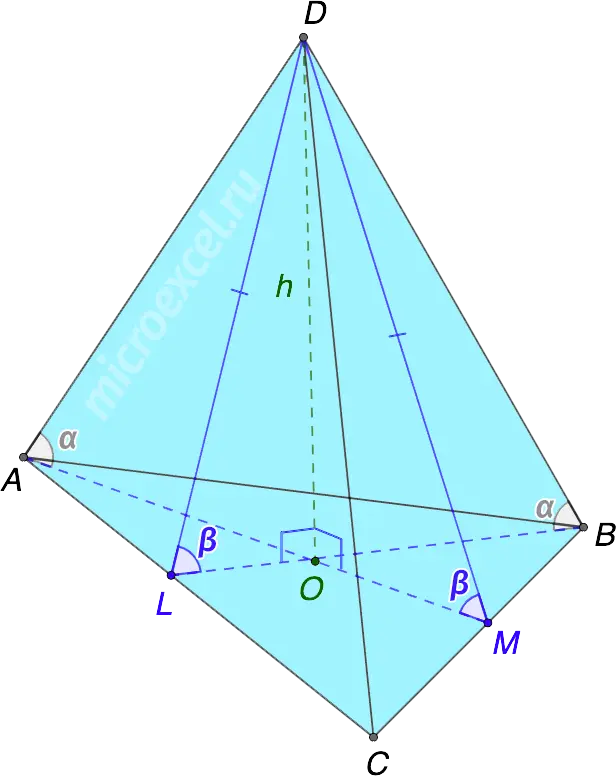
• Base – right / equilateral triangle ABC.
• The side faces are identical isosceles triangles: ADC, BDC и ADB.
• Projection vertices D on the basis – point O, which is the intersection point of the altitudes/medians/bisectors of the triangle ABC.
• DO is the height of the pyramid.
• DL и DM – apothemes, i.e. the heights of the side faces (isosceles triangles). There are three in total (one for each face), but the picture above shows two so as not to overload it.
• ⦟DAM = ⦟ DBL = a (angles between side ribs and base).
• ⦟DLB = ⦟DMA = b (the angles between the side faces and the base plane).
• For such a pyramid, the following relation is true:

  AO:OM = 2:1 or BO:OL = 2:1.

Note: if a regular triangular pyramid has all the edges equal, it is also called correct .

Regular quadrangular pyramid

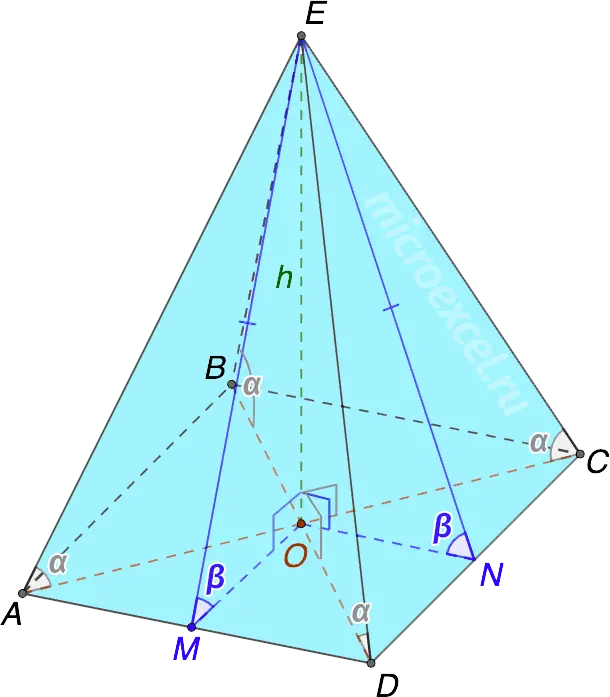
• The base is a regular quadrilateral ABCD, in other words, a square.
• Side faces are equal isosceles triangles: General Conditions of Purchase, BEC, CED и AED.
• Projection vertices E on the basis – point O, is the intersection point of the diagonals of the square ABCD.
• EO – the height of the figure.
• EN и EM – apothemes (there are 4 in total, only two are shown in the figure as an example).
• Equal angles between side edges/faces and the base are indicated by the corresponding letters (a и b).

Regular hexagonal pyramid

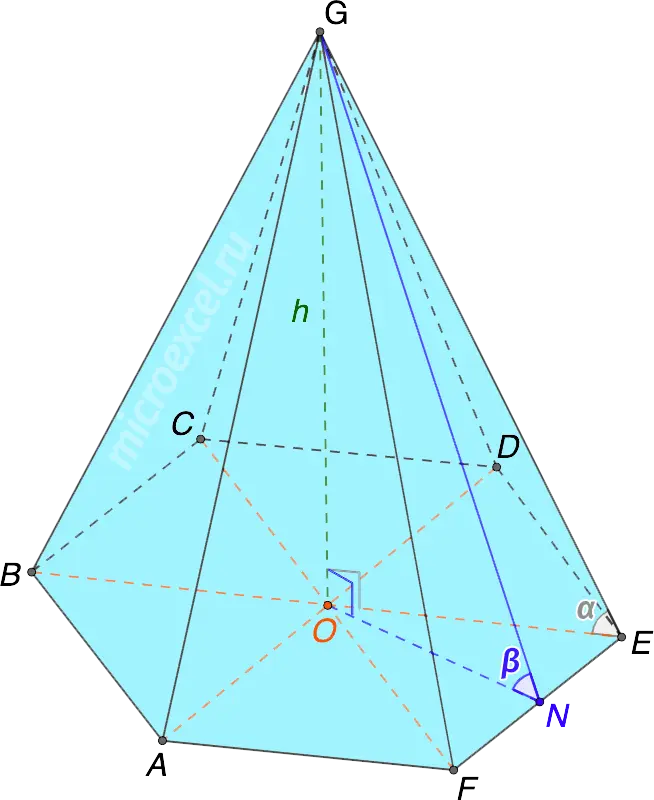
• The base is a regular hexagon ABCDEF.
• Side faces are equal isosceles triangles: AGB, BGC, CGD, DGE, EGF и FGA.
• Projection vertices G on the basis – point O, is the intersection point of the diagonals/bisectors of the hexagon A B C D E F.
• GO is the height of the pyramid.
• GN – apothem (there should be six in total).

Properties of a regular pyramid

1. All side edges of the figure are equal. In other words, the top of the pyramid is at the same distance from all corners of its base.
2. The angle between all side ribs and the base is the same.
3. All faces are inclined to the base at the same angle.
4. The areas of all side faces are equal.
5. All apothems are equal.
6. Around the pyramid can be described, the center of which will be the intersection point of the perpendiculars drawn to the midpoints of the side edges.
7. A sphere can be inscribed in a pyramid, the center of which will be the intersection point of the bisectors, originating in the corners between the side edges and the base of the figure.

Note: Formulas for finding, as well as pyramids, are presented in separate publications.