Ways to Compare Decimals

In this publication, we will look at ways in which you can compare decimal fractions or decimal and ordinary fractions. We will also analyze examples to consolidate the material presented.

Comparison of decimals

1 method

To compare decimals, follow these steps:

1. We equalize the length of both fractions – to the one with fewer decimal places, add zeros at the end (their number depends on how many digits in the fractional part of the “longer” fraction). This action will not change the value of the “short” fraction according to .
2. In turn, we compare the constituent parts of fractions: integers with integers, tenths with tenths, hundredths with hundredths, etc.
3. As soon as one of the parts of one fraction is greater than the same part of the second fraction, this means that it is greater than the other.

Note: A decimal fraction is always greater than a whole natural number if its whole part is equal to the given number. That is:

• 4,3> 4
• 5,46> 5
• 7,017> 7
• etc.

2 method

To compare two decimal fractions, you can from one to the other. If the result is positive (that is, greater than zero), then the minuend is greater than the subtrahend and vice versa (see. Example 2 below).

Comparison of decimal and common fractions

To compare a decimal fraction with an ordinary one, we represent the latter in the form, then we perform the comparison using the methods above.

Or you can do the opposite – convert the decimal to and then already.

Examples

Example 1

Compare decimals 6,4 и 6,45.

Solution

Let’s use the first method. Because in fractions 6,45 two digits after the decimal point, therefore, we are missing in the number 6,4 one sign in the fractional part, and we add zero at the end, resulting in – 6,40.

Now let’s start the comparison:

• The integer parts of the considered fractions are equal: = 6 6.

  So we turn to the comparison of fractional parts.

• Tenths are: = 4 4.

  We move on.

• Judges: 4 <5.

Hundredths of the second fraction is larger, therefore, it is also larger.

Answer: 6,40 <6,45 or 6,4 <6,45.

Example 2

Determine which of the fractions is greater: 5,146 or 5,14.

Solution

Let’s use the second method:

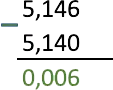
The difference is greater than zero (0,006 > 0), hence, 5,146 > 5,14.

Example 3

Solution

For example, let’s choose the first option:

Now it remains only to compare two decimal fractions: 0,28 и 0,25.

• Whole parts are equal: = 0 0.
• Tenths: = 2 2.
• Judges: 8 > 5.