In this publication, we will consider what a linear inequality is, how it differs from equations, how it is solved. We will also analyze examples on this topic.
Definition of a linear inequality
First, let’s remember the basic mathematical signs used for comparison.
| Symbol | Name | Sign type |
| more | strict sign, i.e. eliminates the number on the border | |
| less | ||
| ≥ | more or equal | non-strict sign, i.e. with a number on the border inclusive |
| ≤ | less or equal |
Linear inequality similar to a linear equation, only instead of the sign “equals” is one of the comparison signs.
Note: unknown variable (usually “X”) is only one and is indicated in the first degree, which is why the inequality is called linear.
For example:
- x + 3 > 7
- 12 – 2x
- x (3 + 5) + 11 ≥ 0
Solving linear inequalities
In general, to solve a linear inequality, we proceed in the same way as when solving equations. That is, we bring the inequality to the following form:
- On the left side, we leave and / or transfer all the unknowns, on the right we collect everything else.
- We divide the right side by the left and get the solution (if required).
Note: when an element is transferred from one part to another, its sign is reversed.
Example 1
x + 3 > 7
x > 7 – 3
x > 4
The resulting solution can be plotted on the number line:
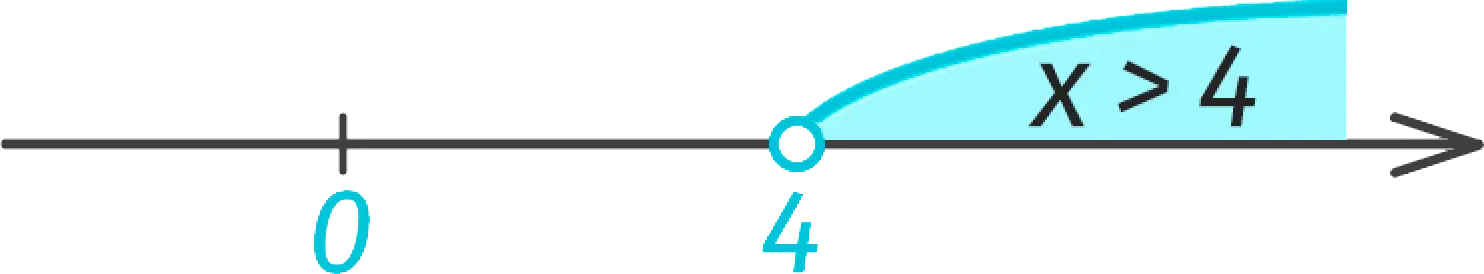
Because strict inequality sign (“more”), the number 4 is marked as an unfilled dot inside. All numbers to the right of four (with the exception of 4) are the solution to our inequality (you can shade or shade).
If the inequality is not strict, then the point inside must be painted over. For example,
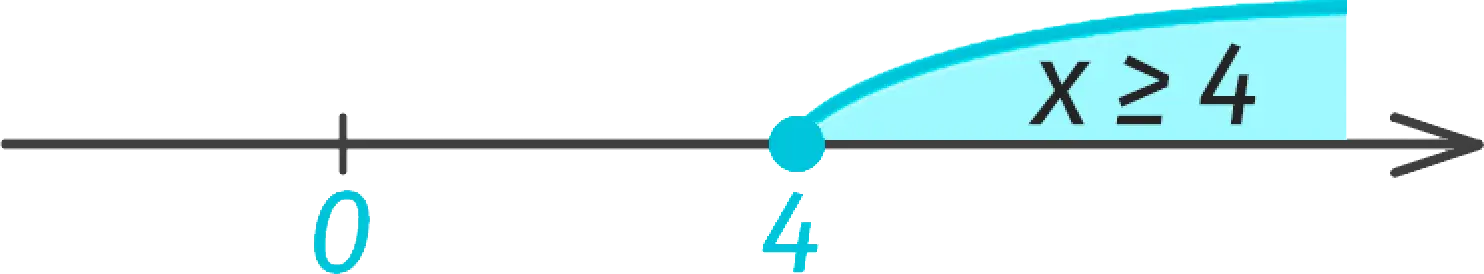
All numbers to the right of 4, including itself, are suitable for us.
In this way, solution inequalities is finding the set of numbers whose substitution into this inequality gives the correct result (that is, these are all values from the shaded / shaded area on the number axis).
Example 2
6x + 8 ≤ 26
6x ≤ 26 – 8
6x ≤ 18
x ≤ 3 (divide both parts by 6)
Note:
When we divide or multiply inequality by some number, then:
- this action is performed for both its parts;
- the inequality sign remains the same if the number is positive, or reversed if it is negative.
Example 3
-2x – 6
-2x
-2x
x > 3 (divide both parts by -2)









