Rectangular coordinate system

In this publication, we will consider what a Cartesian (rectangular) coordinate system is, what elements it consists of, and how the coordinates of an arbitrary point are written in this system.

Defining a Cartesian Coordinate System

Coordinates – this is a kind of exact “address” recorded according to certain rules, at which one or another object can be found.

Coordinate examples:

• geographic latitude and longitude;
• the location of any mark on the numerical axis;
• number of the row and seat on the plane, etc.

When it is required to indicate the coordinates of a point on a plane, the Cartesian (rectangular) system is most often used, named after the French mathematician and physicist Rene Descartes.

System structure:

1. Two straight numerical axes are drawn on the plane, perpendicular to each other (i.e. located at an angle of 90 °). Their intersection at a point O is the origin of coordinates (reference) for each of the axes.
2. The horizontal axis (tends from left to right, as indicated by the corresponding arrow) is called abscissa. It is denoted by the Latin letter “X”, and it is written as “Ox”. The positive part is to the right of the dot “OR”.
3. The vertical axis (tends from bottom to top, which is also indicated by an arrow) is called y-axis. Denoted by letter “Y”, is written as “Ltd”. The positive part is located above the point “OR”.

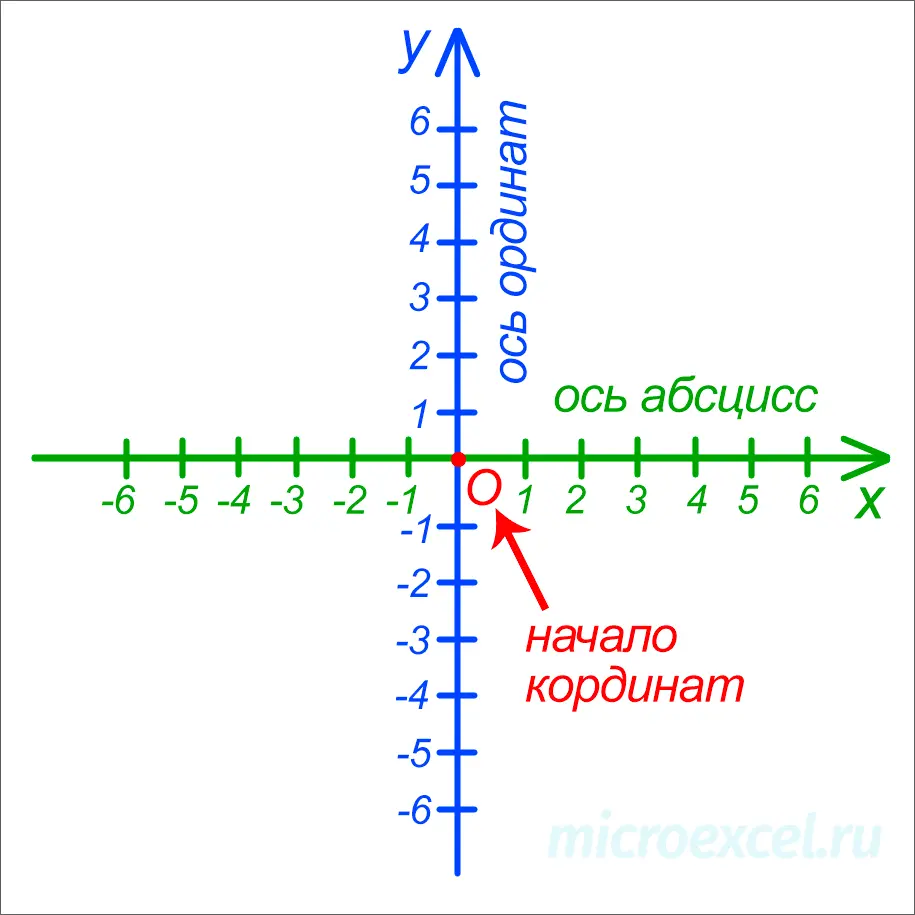
Thus, a rectangular coordinate system includes the following main elements:

1. Coordinate plane is the plane in which the coordinate system is located. Written as “xOy”.
2. Coordinate axes: here is the abscissa (Ox) and here is the ordinate (Oy).
3. Single segments (division price) – distances between dashes on both numerical axes, which are usually equal in length.
4. The values, corresponding to each mark on the axis. Usually written like this:
   • for Ox – from below;
   • for Oy – right or left.
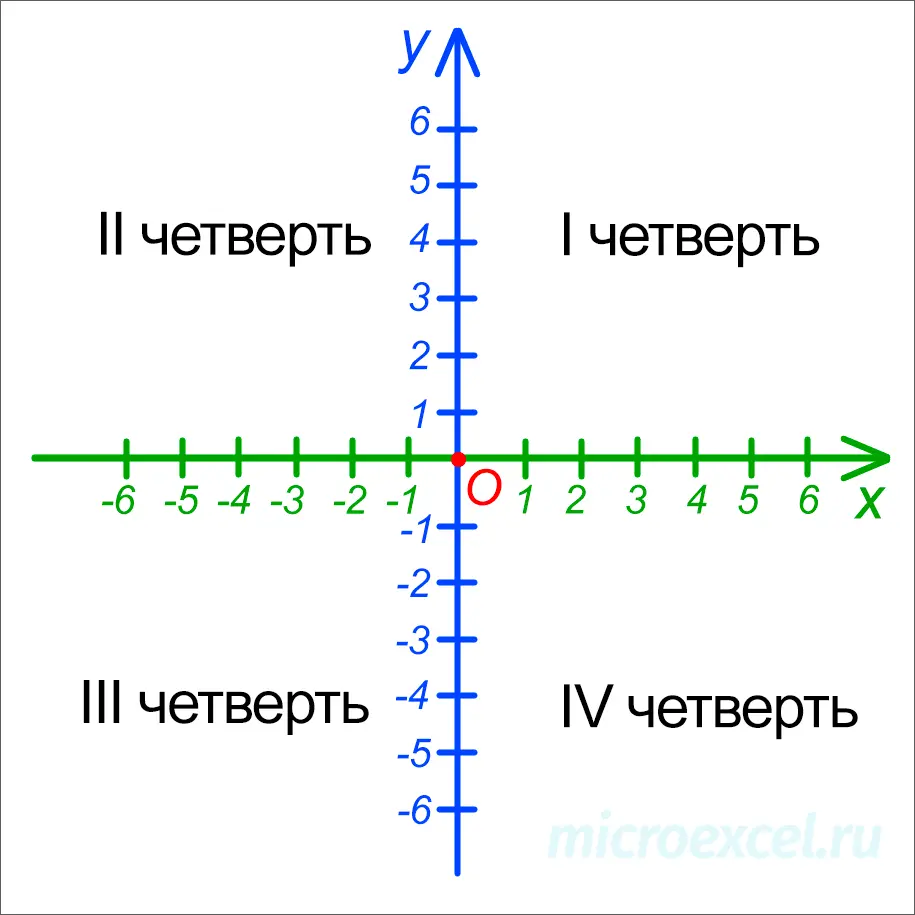
5. Coordinate quarters – zones into which the plane is divided by two axes. Numbered in Roman numerals and arranged as follows:

Recording coordinates

In order to determine the coordinates of an arbitrary point, we lower two perpendiculars from it – one for each axis, and then we calculate the total number of unit segments from the point “OR”.

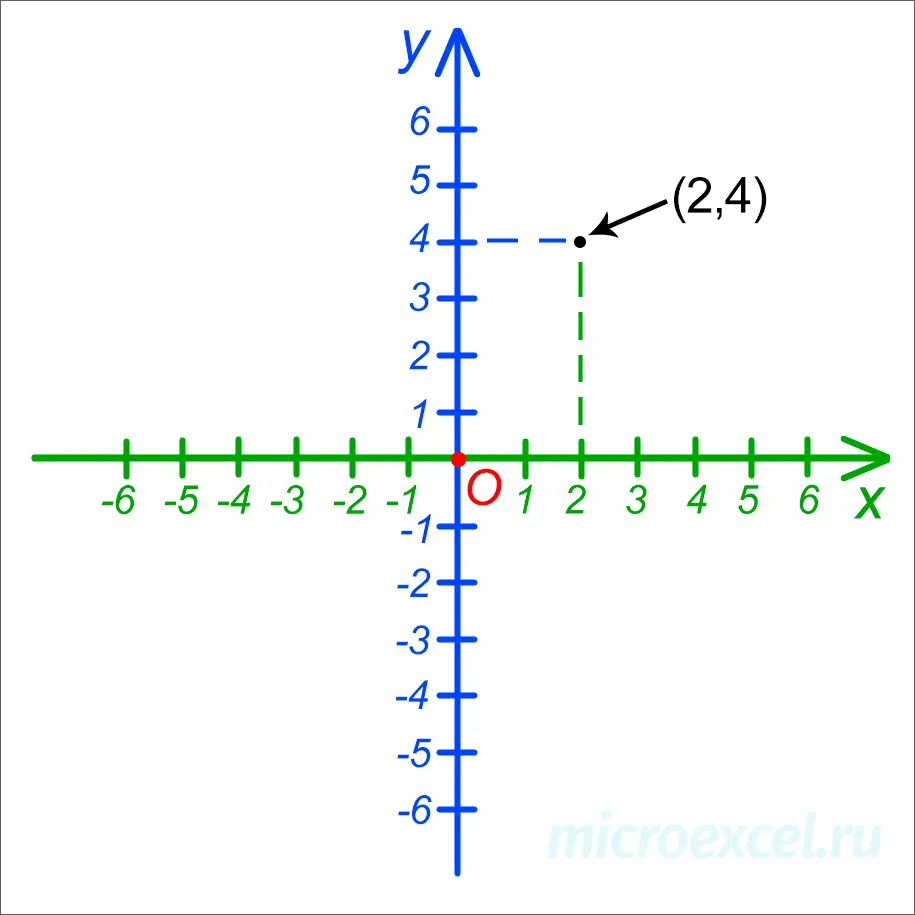
We write the coordinates themselves in brackets, with the first corresponding to the abscissa axis, the second to the ordinate axis. For example, in the figure above, the specified point has coordinates (4,2).