Pythagorean theorem for a right triangle: formula and problems

In this publication, we will consider one of the main theorems of Euclidean geometry, the Pythagorean theorem, which determines the ratio of sides (legs and hypotenuses) in a right triangle, and also learn how to apply it in practice to solve problems.

Theorem formula

In a right triangle, the square of the hypotenuse is equal to the sum of the squares of its legs.

c² = A² + b²

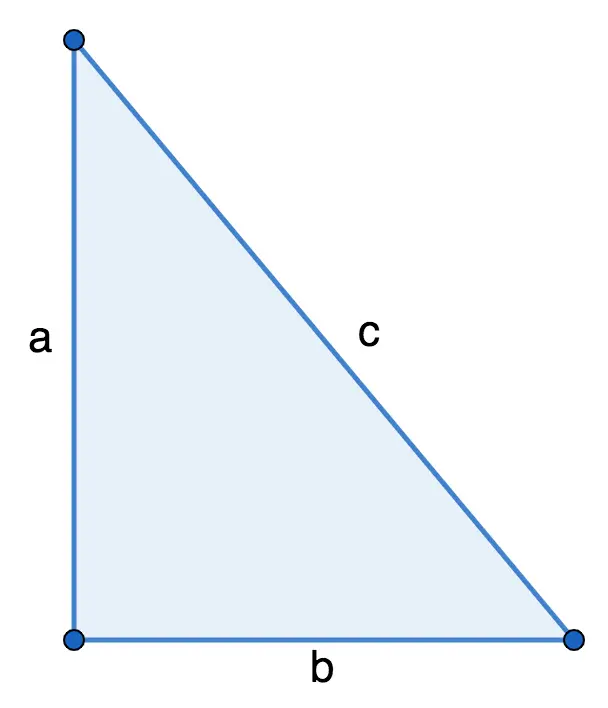
Examples of tasks

Task 1

In a right triangle, one leg is 3 cm, the other is 4 cm. Find the length of its hypotenuse.

Decision:

We use the formula of the theorem: c² = 3² + 4² = 25 cm². Therefore, hypotenuse (c) = 5 cm.

Task 2

One of the legs of a right triangle is 6 cm, and the hypotenuse is 10 cm. Find the length of the second leg.

Decision:

Let’s say 6 cm is the length of the leg a, and you need to find b.

As follows from the formula of the theorem, the square of a leg is equal to the square of the hypotenuse minus the square of the other leg.

That is b² = c² – and² = 10² – 6² = 64 cm². Therefore, b = 8 cm.