Finding the volume of a parallelepiped: formula and tasks

In this publication, we will consider how you can find the volume of a parallelepiped and analyze examples of solving problems to fix the material.

The formula for calculating the volume of a parallelepiped

1. General formula

The volume of any parallelepiped is equal to the product of the area of ​​its base by the height.

V=S_main ⋅ h

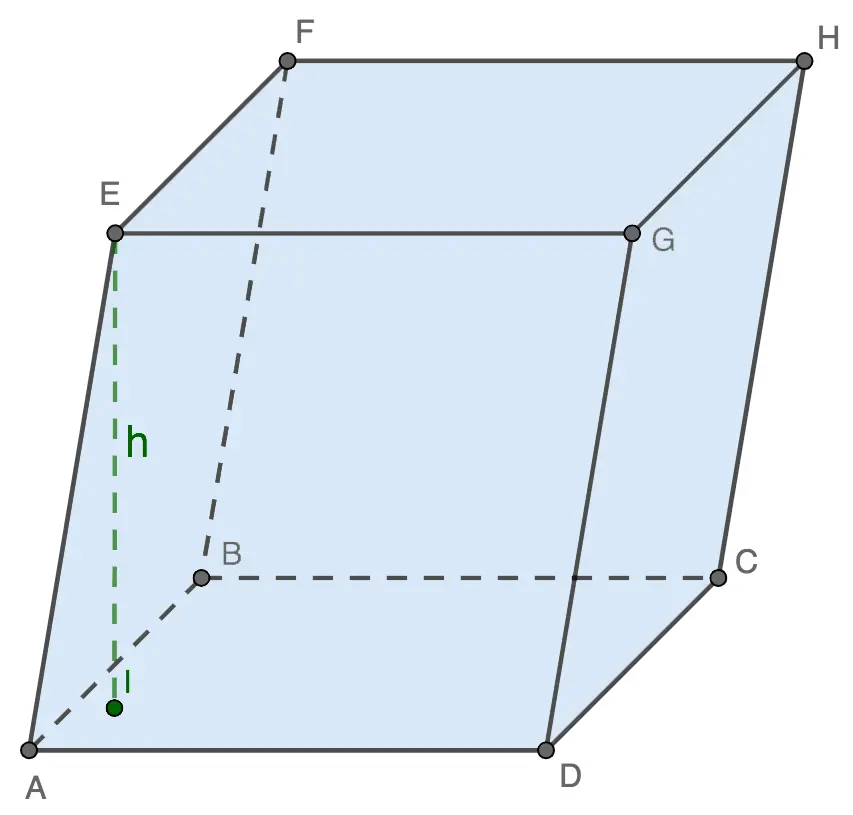
• S_main – base area (ABCD or EFHG, equal to each other);
• h – height.

This formula is valid for all types of geometric shapes:

• oblique – side faces are not perpendicular to the bases;
• straight – all side faces (4 pieces) are rectangles;
• rectangular – all faces (side and base) are rectangles;
• rhombohedron – all faces are equal rhombuses;
• Cuba All faces are equal squares.

2. Volume of a rectangular parallelepiped

The volume of a figure is equal to the product of its length times its width times its height.

V = a ⋅ b ⋅ c

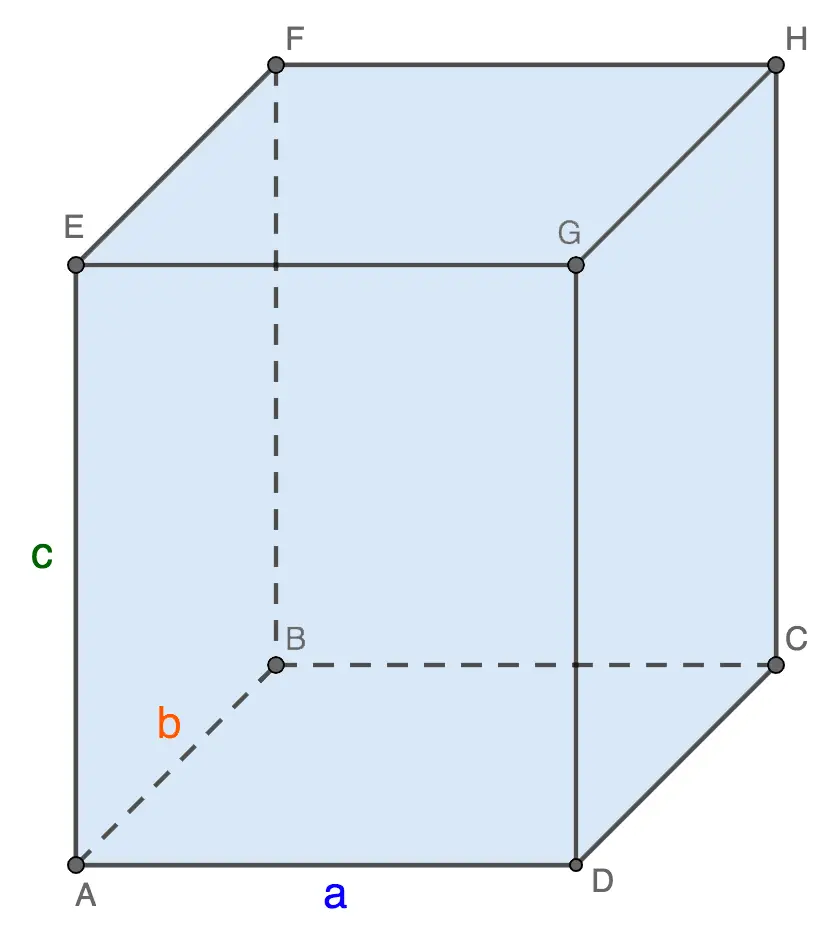
The formula follows from the following statements:

• The base of the figure is a rectangle whose area is calculated as the product of its length (a) to the width (b).
• The height of the figure is the length of the side face (c).

Examples of tasks

Task 1

Find the volume of the parallelepiped if it is known that the area of ​​its base is 20 cm²and the height is 7 cm.

Decision:

We use the first formula, substituting the values ​​​​known to us into it:

V=20cm² ⋅ 7 cm = 140 cm³.

Task 2

Given a rectangular parallelepiped. The length and width of its base are 9 cm and 5 cm, respectively, and its height is 6 cm. Find the volume of the figure.

Decision:

Let’s use the formula for this type of figure:

V = 9 cm ⋅ 5 cm ⋅ 6 cm = 270 cm³.