Contents
In this publication, we will consider how to calculate the volume of a right circular cone and analyze examples of solving problems.
Volume formula
1. Through the base area and height
The volume (V) of a cone is equal to one third of the product of its height and the base area:
![]()
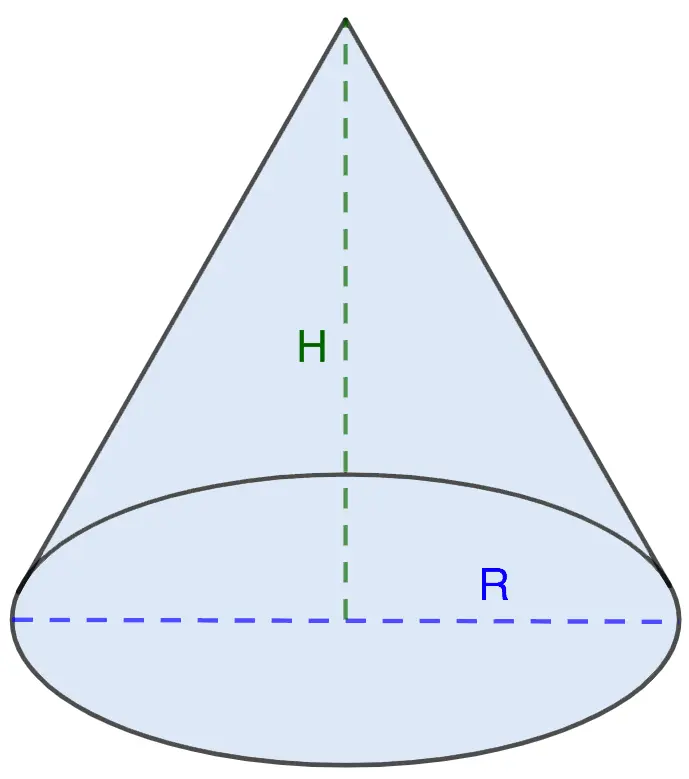
2. Through the base radius and height
As we know, the base of the cone is a circle, the area of uXNUMXbuXNUMXbwhich is calculated by the formula: S = πR2.
Therefore, the formula for calculating the volume of a cone can be represented as:
![]()
Those. The volume of a cone is equal to one third of the product of its height and the number π and the radius of the base squared.
Note: in calculations the value of the number π rounded up to 3,14.
The formula for finding the volume of a truncated cone is presented in a separate.
Examples of tasks
Task 1
Find the volume of a cone if the area of its base is known – 50,24 cm2, and also, height – 9 cm.
Decision:
Let’s apply the first formula by substituting the given values into it:
![]()
Task 2
The height of the cone is 7 cm, and its radius is 3 cm. Find the volume of the figure.
Decision:
Using the second, more extended formula, we get:
![]()









