Contents
In this publication, we will consider formulas with which you can calculate the radius of a circle inscribed in a square. We will also analyze examples of solving problems to consolidate the theoretical material.
Formulas for calculating the radius of an inscribed circle
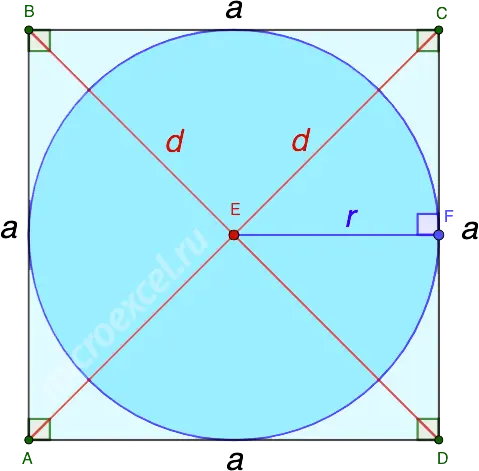
Through the side of the square
Radius r A circle inscribed in a square is half the length of its side. a.
![]()
Through the diagonal of the square
Radius r a circle inscribed in a square is equal to the length of its diagonal ddivided by the product of 2 and the square root of XNUMX.
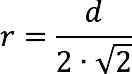
Examples of tasks
Task 1
Find the radius of a circle inscribed in a square if it is known that the length of its side is 7 cm.
Solution
Let’s use the first formula, substituting the known value into it:
![]()
Task 2
It is known that the radius of a circle inscribed in a square is 12 cm. Find the length of its diagonal.
Solution
The formula for finding the diagonal can be derived from the formula for calculating the radius of a circle:
![]()









