Contents
convex quadrilateral – This is a geometric figure obtained by connecting four points on a plane that should not lie on one straight line. In this case, the sides formed in this way should not intersect.
Area formula
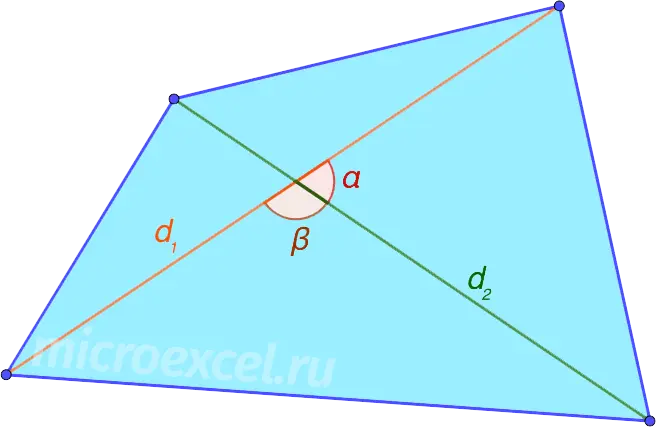
Along the diagonals and the angle between them
Area (S) of a convex quadrilateral is equal to one second (half) of the product of its diagonals and the sine of the angle between them.
![]()
On four sides (Brahmagupta’s formula)
To use the formula, you need to know the lengths of all sides of the figure. It should also be possible to describe a circle around the quadrilateral.
![]()
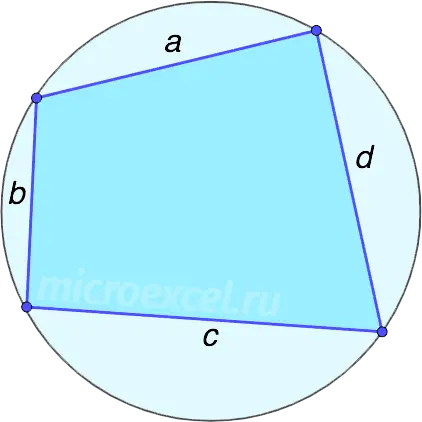
p – semi-perimeter, calculated as follows:
![]()
Along the radius of the inscribed circle and sides
If a circle can be inscribed in a quadrilateral, its area can be calculated using the formula:
S = p ⋅ r
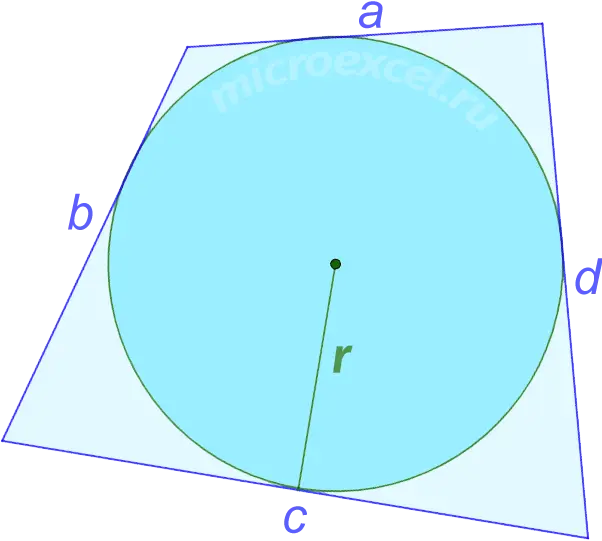
r is the radius of the circle.
Example of a problem
Find the area of a convex quadrilateral if its diagonals are 5 cm and 9 cm and the angle between them is 30°.
Decision:
We substitute the values u1bu2bknown to us into the formula and get: S u5d 9/30 * 11,25 cm * XNUMX cm * sin XNUMX ° uXNUMXd XNUMX cm2.









