Collinear vectors

In this publication, we will consider which vectors are called collinear and list the conditions under which they are. We will also analyze examples of solving problems on this topic.

Conditions for collinear vectors

Vectors that lie on one or more parallel lines are called collinear.

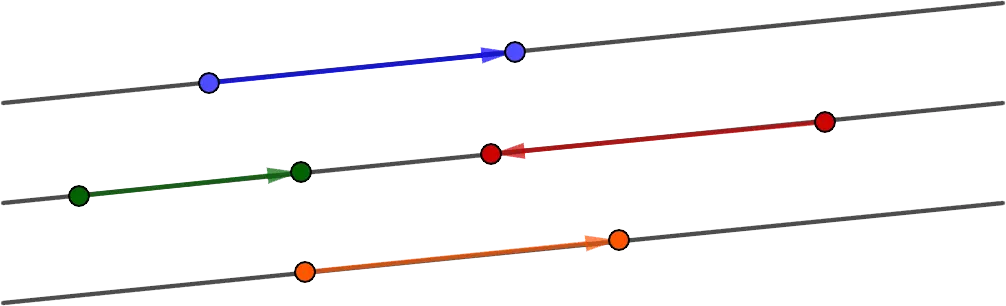
Two vectors are collinear if one of the following conditions is true:

1. There is such a number nat which a · n = b.

2. The ratios of the coordinates of the vectors are equal. But this condition cannot be applied if one of the coordinates is equal to zero.

3. The cross product is equal to the zero vector (applicable only for three-dimensional problems).

Examples of tasks

Task 1

Given vectors a = {2; 5}, b = {3; 7} и c = {6; 15}. Let us determine whether any of them are collinear.

Decision:

The given vectors do not have zero coordinates, so we can apply the second collinearity condition.

Therefore, only the vectors are collinear a и c.

Task 2

Let us find out for what value n vectors a = {4; 10} и b = {2; n} collinear.

Decision:

Because there are no zeros among the coordinates, according to the second condition, we can make up their ratio in order to calculate the missing element.

So n = 2 10 : 4 = 5.