Arithmetical progression – this is a numerical sequence in which, starting from the second number, each subsequent number is equal to the previous one plus a constant term.
General view of an arithmetic progression
a1,1 + d, a1 + 2d, … a1 + (n – 1) d, …
d – step or difference of progression; this is the constant term.
Members of the progression:
- a1
- a2 = A1 + d
- a3 = A2 + d = a1 + 2d
- etc.
The numbers 1,2,3 … are their serial numbers, i.e. the place they occupy in the sequence.
Properties and formulas of arithmetic progression
1. Finding the common nth term (an)
- an = An-1 + d
- an = A1 + (n – 1) d
- an = Am – (m – n) d
2. Progression difference
d = an – andn-1
The following formula is also used to find the step:
![]()
3. Characteristic property
Sequence of numbers a1,2,3 … is an arithmetic progression if the following condition is satisfied for any of its members:
![]()
4. The sum of the first terms of the progression
To find the sum of the first terms of an arithmetic progression, you must use the formula:
![]()
- n is the number of summed terms.
If an replaced by a1 + (n – 1) d, then you get:
![]()
5. The sum of the members of the progression from the nth to the mth
![]()
- (m – n + 1) is the number of summed terms.
If am replaced by an + (m – n) d, then we get:
![]()
6. Convergence of progression
The arithmetic progression converges when d = 0, in all other cases it diverges.
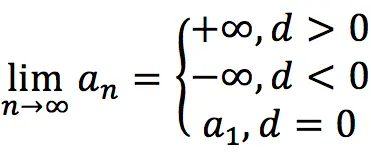
In this case, if:
- d > 0, the progression is called increasing;
- d – decreasing;
- d = 0 – stationary.









