Ivan Limonchenko, Head of the International Laboratory for Algebraic Topology and Its Applications, Faculty of Computer Science, Higher School of Economics, spoke about Dennis Sullivan, who received the Abel Prize in 2022
As you know, the Nobel Prize is not awarded either in mathematics or in computer science that has grown out of it. No one knows the reasons for this, but there is a legend that Alfred Nobel’s wife left him for a mathematician. Of course, this is nothing more than an anecdote, if only because Nobel had no wife.
There is another version according to which Nobel did not recognize the value of mathematics as an engine of progress – which is ironic, since in the XNUMXth century it was mathematics that played a fundamental role in the development of physics, computer science and economics.
However, a mathematician can only qualify for the Abel Prize or the Fields Prize (which is awarded to scientists under 40), and a computer scientist for the Turing Prize.
What is topology?
Topology is even higher than higher geometry: it is post-geometry, a science that studies the properties of an object that do not change during its continuous deformations. What does this actually mean? The figures with which topology works can be considered as if they were made of plasticine.
Imagine a plasticine donut, donut or “solid torus”: it is easy to change its shape without resorting to tears and gluing (“continuously deform”), and turn it into a mug, as well as a weight, therefore, from the point of view of topology, a weight, a mug and a bagel are the same topological space (more precisely, they are “topologically equivalent” spaces). Thus, such characteristics as length, width, height of a figure do not matter for topology. Topologically equivalent to each other will be a triangle, a square and a circle of any size: from the point of view of topology, all their properties are the same, since they are flat and they have no holes.
At the same time, a pretzel (it has two holes) cannot be continuously deformed into a donut (it has one hole), and a donut cannot be deformed into a bun, or a “three-dimensional” disk (it has no holes). These figures have a different number of holes, and you cannot turn one into another simply by “rolling it out” (without tears and gluing). For their surfaces, the number of holes is a topological invariant that plays a key role in their classification; these “two-dimensional” surfaces are not topologically equivalent, that is, from the point of view of topology, they are different topological spaces.
Another fundamental topological invariant of a “manifold” (that is, a surface of an arbitrary number of dimensions) is its dimension. This is the number of independent coordinates that can be used to set the position of any point on it: for example, the surface of the Earth is two-dimensional, since the position of any point on it is described by two numbers – latitude and longitude (this is an example of a “closed manifold”: it is limited in space and has no edge) , but the bun is already three-dimensional, since the position of any point in it is described by three numbers: the distance to the center of the ball, latitude and longitude on the sphere with the center in the center of the ball on which it lies (this is an example of an “open manifold”: it is limited in space, but it has an edge, which is its surface – a two-dimensional sphere).
By the way, the “millennium problem”, which Grigory Perelman solved, is also from the field of topology. According to it, any simply connected closed three-dimensional manifold is topologically equivalent to a three-dimensional sphere – in other words, any bounded “three-dimensional” surface that does not have edges and holes is topologically equivalent to the surface of a “four-dimensional” kolobok (it lives in four-dimensional space: we can assume that to three dimensions, in which we live, one more thing is added – time).
Why topology is needed
It is remarkable that, from a topological point of view, one can work not only with the most diverse polyhedra, such as a cube, but also with graphs, various complex surfaces, and also study the decoupling of knots and decoupling of links – all this opens up wide horizons and prospects both for the development of the theory , and for practical applications of topology.
Today, the ideas and methods of topology are used in data analysis, machine learning, robotics, and even to study the brain — all these modern areas of topology applications are being actively developed in our laboratory at the HSE Faculty of Computer Science.
Recently, topology has increasingly penetrated into physics, chemistry, and biology. Topology is associated with DNA research. It also allows you to explore and describe spatial relationships, which can also help you model clothes. For example, it will be possible to create various effects due to which clothes will look the most advantageous (stretching, narrowing the figure), as well as to optimize the process of making things, minimizing the time and resources spent on cutting and sewing.
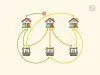
There are many different visual problems that have a topological nature: for example, an observation that goes back to Euler – “the problem of three houses and three wells”: it is impossible to connect each of the three houses with each of the three wells by non-self-intersecting paths, so that all paths do not intersect in pairs . Here the matter takes place on a plane: of course, in space this is easy to do; a little more difficult, but you can also do it on the surface of the mug (on the “torus”).

There is also a theorem “about combing a hedgehog”: it is impossible to comb a hedgehog curled up in such a way that not a single needle sticks out of it. In the language of topology, the same result is formulated as follows: “there is no non-zero continuous tangent vector field on a two-dimensional sphere.”

Who is Dennis Sullivan
The Abel Prize, like the Nobel Prize, is awarded not for one achievement, but for the work of a scientist as a whole. Dennis Sullivan was named the laureate “for his pioneering contributions to topology in its broadest sense, and in particular its algebraic, geometrical and dynamical aspects”.
Dennis Sullivan (in Soviet and Russian scientific journalism his surname is spelled as Sullivan) is an outstanding specialist in the field of algebraic and geometric topology, and the theory of dynamical systems. He is currently the Albert Einstein Chair at the City University of New York, a professor at Stony Brook University, and a member of the US National Academy of Sciences. He is the recipient of a number of prestigious awards, including the Wolf Prize in Mathematics (2010) and now the Abel Prize (2022).
Sullivan received his PhD from Princeton University (1966) under William Browder. In his dissertation, Sullivan studied the following classical topology conjecture: if two multidimensional smooth surfaces (“smooth manifolds”) are topologically equivalent (“homeomorphic”), that is, all their topological properties are the same, then they are smoothly equivalent (“diffeomorphic”). Using the analogy with plasticine figures, smooth figures can be deformed without creating gaps and glues, as well as corners. In the general case, this conjecture is false, as was shown in 1956 by another eminent topologist, winner of the Fields Prize (1962) and Abel Prize (2011), John Milnor. However, Sullivan succeeded in proving that, under some technical restriction imposed on the topology of simply connected smooth manifolds, this conjecture holds in dimensions five and higher.
His research
Sullivan is one of the creators of the method of surgery, the main method of modern differential topology. Surgery (or “restructuring”) of a manifold consists in cutting out a piece of the surface and gluing in its place along the common edge of a piece of another manifold, so that the properties of this surface will change according to known rules. For example, if we cut out a circle on the surface of a donut and glue exactly the same surface along its boundary (circle) (it is called a “handle” in differential topology), then we get the surface of a pretzel, and if we glue a Möbius strip to a sphere with a hole, we get projective plane. So a mug is obtained from a glass by gluing a handle both from the point of view of everyday life and from the point of view of topology.
Together with Daniel Quillen, they created a rational theory of homotopies, which studies a class of “formal” topological spaces, arranged quite simply from the point of view of calculating their algebraic-topological invariants: they ignore all possible torsion.
Together with his wife, the mathematician Moira Ches, Sullivan created another new branch of topology, string topology, which studies algebraic structures on homology spaces of free loops. At present, string topology is used to solve the fundamental problem of constructing topological quantum field theories in mathematical physics. By the way, Moira Ches has an interesting hobby: to materialize abstract concepts, she crochet complex topological shapes.
Sullivan is also interested in the turbulent behavior of fluids, such as water flows. According to him, he dreams of discovering patterns that could make such a movement predictable on a large scale.










